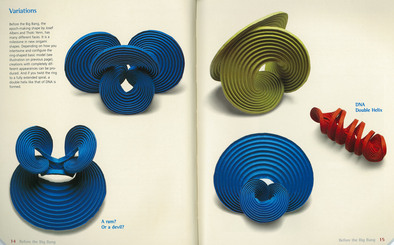
There is a surprisingly old history to curved-crease sculpture,
going back to the 1920s at the Bauhaus.
We give here a partial history focusing on the earliest known references.
|
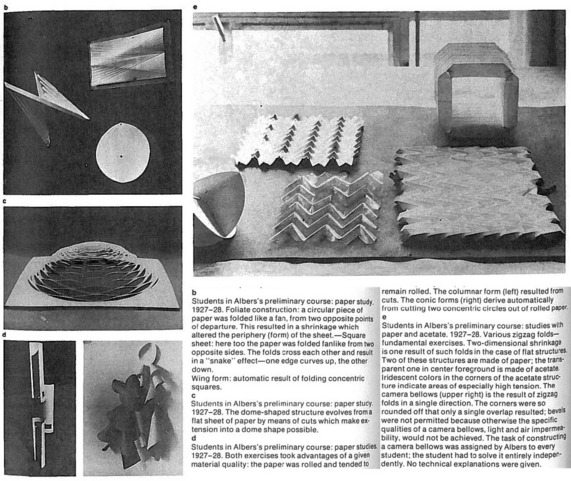
Of interest to origamists are other foldings (with straight creases)
created in 1927–1928 Albers' class: much earlier models
similar to Miura-ori and Fujimoto folds,
and the earliest known hyperbolic paraboloid.
The image on the right is from page 435 of
Bauhaus: Weimar, Dessau, Berlin, Chicago.
The hyperbolic paraboloid or hypar
(shown in the top left) is similar in spirit to the concentric circle model.
Take a square piece of paper and fold concentric squares, alternating
mountain and valley, and also fold the diagonals.
Again the paper pops automatically into a saddle curve;
this time, however, it appears to be a mathematical surface
called the hyperbolic paraboloid.
Hannes Beckmann
writes
in his 1970 article “Formative Years”
[in Bauhaus and Bauhaus People, edited by Eckhard Neumann,
New York: Van Nostrand Reinhold, 1970, page 196]:
I remember vividly the first day of the [Preliminary Course]. Josef
Albers entered the room, carrying with him a bunch of newspapers. … [and]
then addressed us … “Ladies and gentlemen, we are poor, not rich. We can't
afford to waste materials or time. … All art starts with a material, and
therefore we have first to investigate what our material can do. So, at the
beginning we will experiment without aiming at making a product. At the
moment we prefer cleverness to beauty. … Our studies should lead to
constructive thinking. … I want you now to take the newspapers … and try
to make something out of them that is more than you have now. I want you to
respect the material and use it in a way that makes sense — preserve its
inherent characteristics. If you can do without tools like knives and
scissors, and without glue, [all] the better.”
|
|
|
The title “Computational Origami” refers to our underlying
algorithmic goal of determining the mathematical curved surface that
results from different kinds of pleated folding.
This kind of “self-folding origami” may have applications to
deployable structures that can compress very small by folding tightly
and later relax into its natural curved form.
To control this process, we must understand what forms result from
different pleatings, and how to design pleatings that make desired forms.
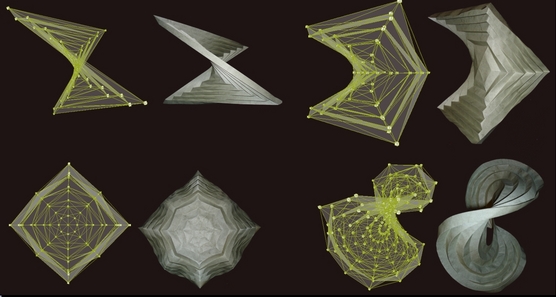
With MIT student Jenna Fizel (2004–2006), we have
developed software to simulate the physics of pleated paper folding.
These simulations accurately reproduce the observed physical behavior.
The image on the right shows four examples (first showing the simulation,
then a real model of folded paper): the hyperbolic paraboloid,
concentric hexagons, concentric octagons, and concentric circles.
We are currently building larger sculptures by joining many
smaller elements with various types of curves.
See our curved-crease sculpture.
|
|



.jpeg)